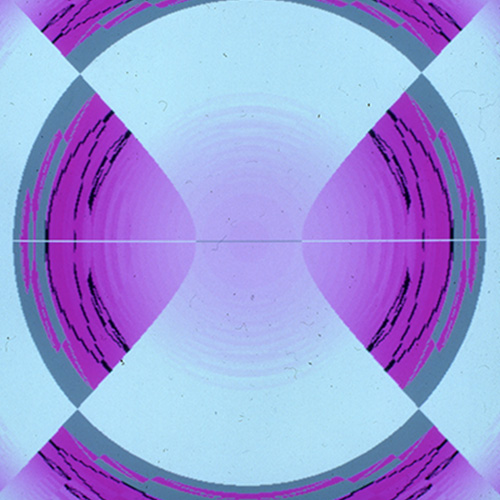



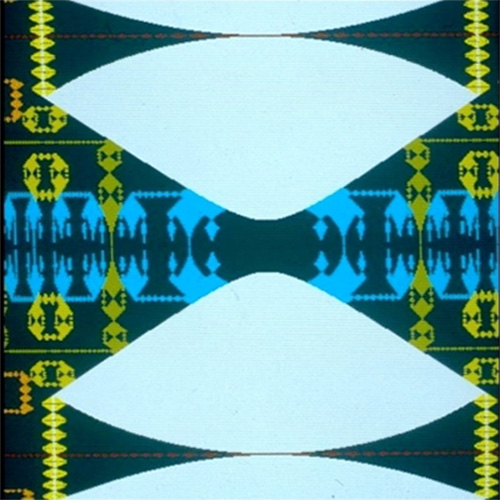





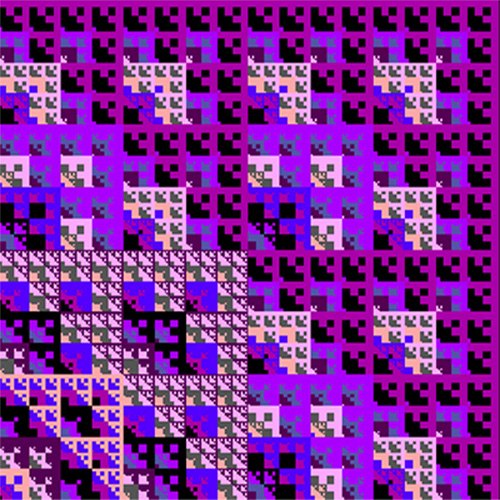

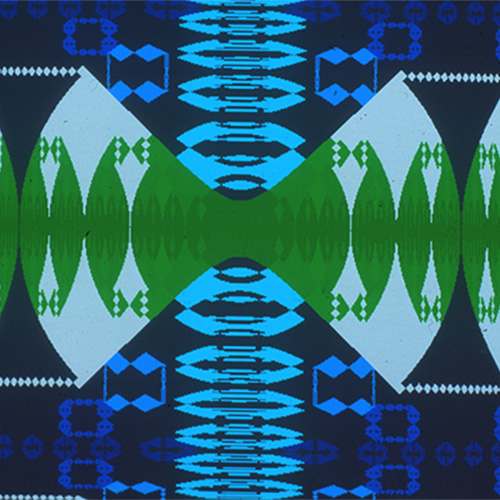

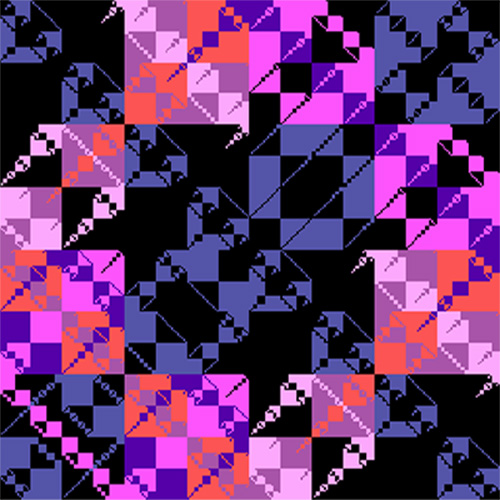

Logics Series (1980-1995)
Logics is a prerequisite for anyone who studies mathematics. But logic is also required in the language we use to converse. Curiously, it is extremely difficult to precisely define logic mathematically, even though it works in an apparently simple way. It was the Viennese philosopher and mathematician Kurt Goedel who was the first to establish that this is a very fundamental problem with which we associate space and time in everyday language. At first sight, it seems quite natural to us to link such fundamental aspects as space and time with logic. Because a” before and after” relationship or a “if this here, then that there” are causally dependent on space and time. Logic is therefore linked to space and time in human thinking. Additionally, we always have a clear opinion when a statement is “true” or “false”. But how can all this be described algebraically in an exact way, in a formalized logic with the different possible links “and”, “or”, “not”? The answers belong to the most difficult problems of Boolean algebra.


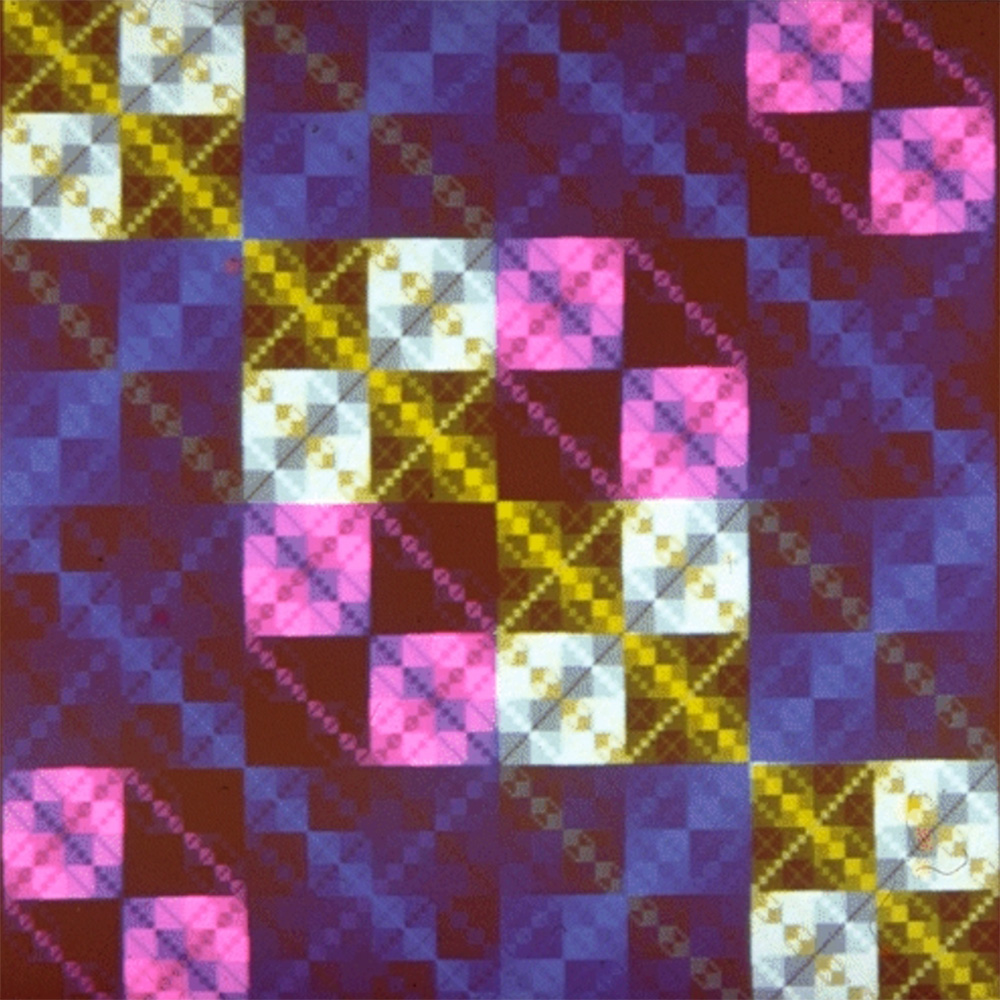



With the Logics series, Herbert W. Franke set out into the world of “cold logic” and its connections in order to explore this important special area of mathematics visually. The resulting worlds show a variety of forms, which are given an additional appeal by the different color combinations chosen.
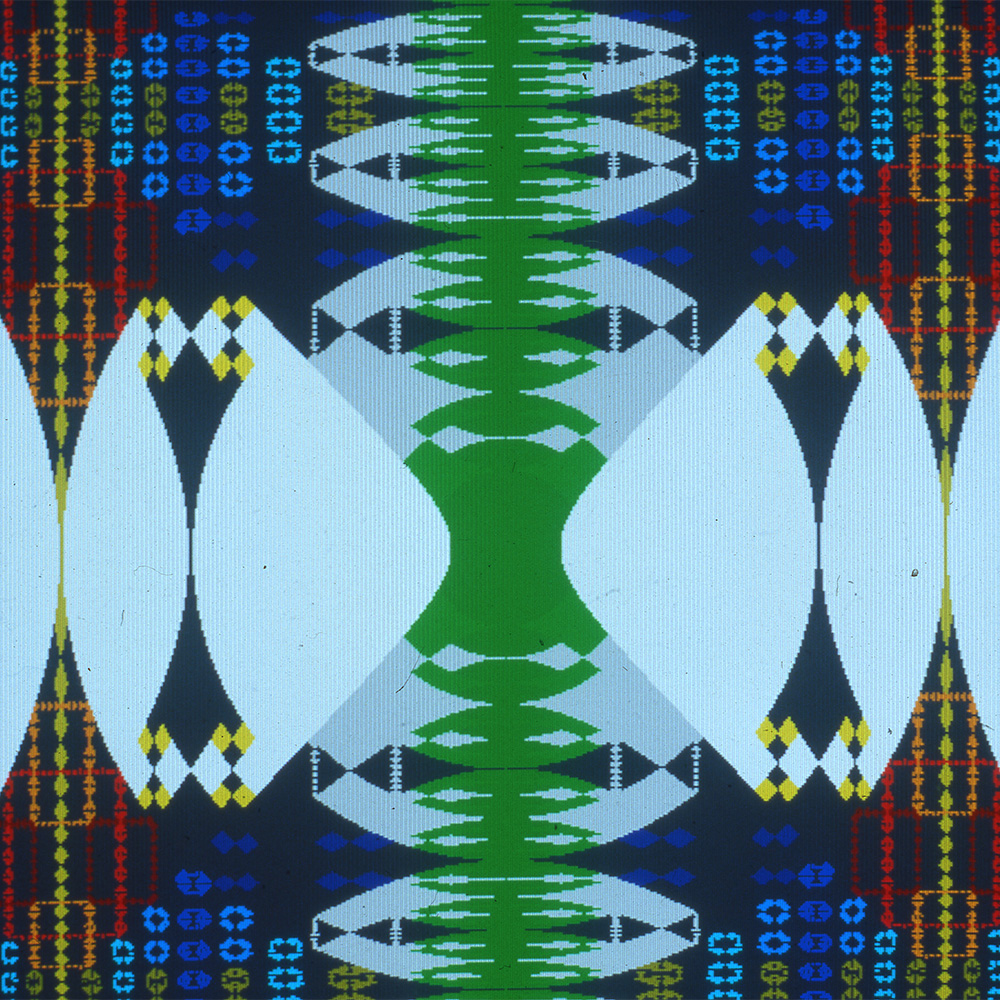

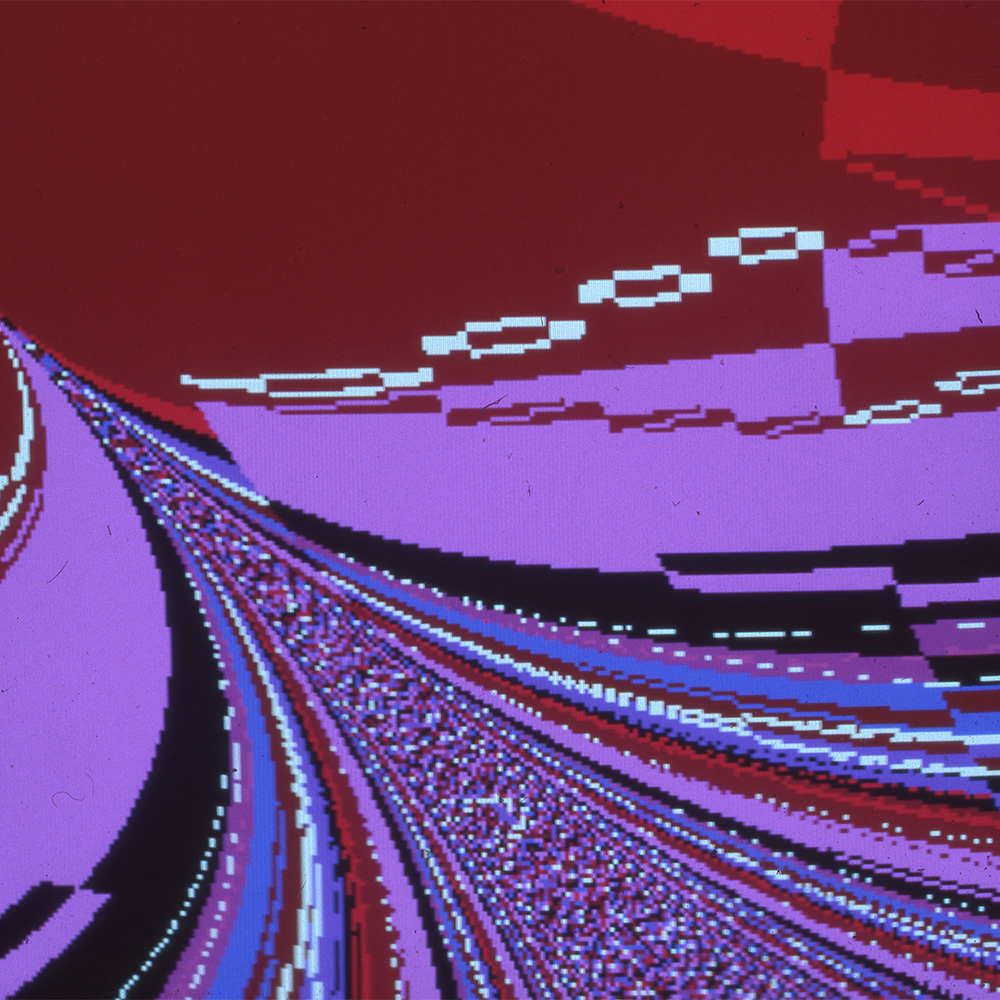



The term Math Art summarizes the results of a long series of experiments that Herbert W. Franke carried out together with the physicist and programmer Horst Helbig from 1980 onwards. It was about the use of mathematical methods, which were not developed and used for scientific purposes, but for aesthetic purposes. The main goal of the 15-year project (1980-1995) was the investigation of numerous mathematical disciplines regarding their aesthetic dimension and the visualization of complex mathematical relationships from algebraic formulas to stochastic relationships. In the course of the work, not only was the aesthetic dimension of formulas and functions explored, but a whole series of new graphic routines were also created, which were integrated into the software DIBIAS (for digital ipicture processing system) as a fixed component, including representations in 2D and 3D as well as further processing with the methods of image transformation (picture processing). The heart of the computer was a Comtal Image Processing System and the software DIBIAS with a resolution of up to 2048 x 2048 pixels and around 16 million colors.
Click here for the other series of Math Art:
Serie Algebra
Serie Buchstaben
Serie Bühnenbilder
Serie Felder
Serie Fourier-Transformationen
Serie Fraktale
Serie Komplexe Zahlen
Serie Logik
Serie Picture Processing
Serie Zufall
